Limits, schlimits: It’s time to rethink how we teach calculus, Ars Technica

        

Calculus has a formidable reputation as being difficult and / or unpleasant, but it doesn’t have to be. Bringing humor and a sense of play to the topic can go a long way toward demystifying it. That’s the goal of math teacher Ben Orlin’s new book,Change Is the Only Constant: The Wisdom of Calculus in a Madcap World,a colorful collection of 28 mathematical tales connecting concepts in calculus to art, literature, and all manner of things human beings grapple with on a daily basis.

His first book,Math with Bad Drawings, afterOrlin’s blogof the same name, was published last year. It included such highlights as placing a discussion of thecorrelation coefficientand “Anscombe’s Quartet“into the world of Harry Potter and arguing that building the Death Star in the shape of a sphere may not have been Darth Vader’s wisest move.We declared it“a great, entertaining read for neophytes and math fans alike, because Orlin excels at finding novel ways to connect the math to real-world problems — or in the case of the Death Star, to problems in fictional worlds. ” And now, he has taken on the challenge of conveying the usefulness and beauty of calculus with tall tales, witty asides, and even more bad drawings.

Calculus boils down to two fundamental ideas: the derivative, which is a way of measuring instantaneous change, and the integral, which describes the accumulation of an infinite number of tiny pieces that add up to a whole. “The derivative is all about isolating a single moment in time, and the integral is all about gathering together an infinite stream of moments to develop a holistic picture,” Orlin told Ars.

Ilike to thinkof the derivative and the integral as the two ends of a hammer: one is for pounding in the nails, and the other is for pulling them out. The first is a process of subtraction and division; the second, a process of multiplication and addition. Each “undoes” the work of the other. And thefundamental theorem of calculusmakes it possible to change one problem into another problem. For instance, if we have an equation that tells us the position of a falling apple, from that we can deduce the equation for the velocity of the apple at any given moment of its fall.

If calculus is so simple and straightforward, why does it strike fear and trepidation into the hearts of so many ? It likely has something to do with the way in which it is traditionally taught. The experience of writingChange Is the Only Constanthelped Orlin refine his ideas on how one might move away from longstanding dogma and rethink that traditional approach.

That said, while there are teaching notes included as appendices, this is not a textbook. “The book doesn’t do much in the way of computation,” he said. “It’s more about telling stories about the concepts and applications.” A Sherlock Holmes story, for instance, demonstrates how to use tangent lines to figure out which direction a bicycle was traveling from the tracks left in the mud.

Ars Technica:Why did you choose to write about calculus for your second book?

(Ben Orlin) : I was excited about calculus before I had an idea of ​​how to tell the story. The first inklings of this book were probably back around 2012. I was working on a book that I calledThe Riemann Calculus Textbook, written in Dr. Seuss style rhymes. It didn’t quite pan out. It might have been okay as a companion text for a course, but it wasn’t really a good way of popularizing calculus, because it was just too slavishly following the way the material is presented in a course.

Then I had a different approach: a book I calledThe Poet’s Calculus, which was going to connect each idea in calculus to a different connection in the humanities. So the poetry of (Adrienne Richwould be a metaphor for limits, and the paintings ofEdgar Degaswould be connected to derivatives of motion, velocity, and acceleration. But that was a little too over-conceptualized. There were about three or four chapters that really worked and then the rest were pretty strange connections.

Finally, I decided to build it around all my favorite stories that touched on calculus, stories that get passed around in the faculty lounge, or the things that the professor mentions off-hand during a lecture. I realized that all those little bits of folklore tapped into something that really excited me about calculus. They have a time-tested quality to them where they’ve been told and retold, like an old folk song that has been sharpened over time.

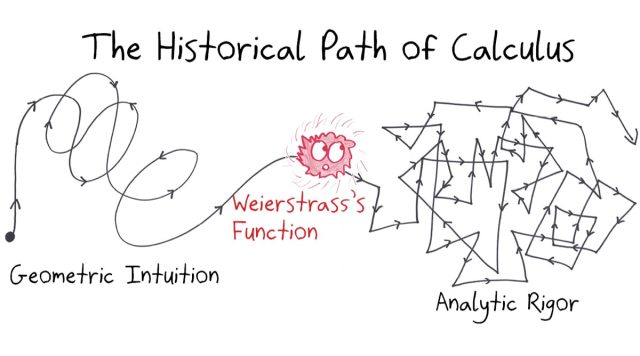
Ars: You write that you found yourself moving away from the dogma of how calculus is traditionally taught — for example, not teachinglimitsfirst and foremost. What are your thoughts on how we can better teach calculus?

(Orlin) : The way we teach calculus is shaped by two considerations. One is that we use calculus as a gatekeeping course very often. So even students who won’t in their careers need calculus, wind up having to pass through it as this gauntlet to gain access to these selective educational opportunities. For that purpose as gatekeeper, we tend to really play up the pencil and paper, computational problem-solving aspects of it. To be fair, that’s an important aspect of calculus; you need to move through in a certain sequence. It’s very clear you need to do derivatives before integrals, because to take an integral is to take an antiderivative, and that’s a harder process. That winds up putting pretty firm constraints on how you can sequence things.

Since the early to mid – 20 th century, teaching calculus has also been driven by the desire for a rigorous axiomatic development— that is, wanting to start from first principles and make sure every theorem is proved and that every rule is justified in a way that is satisfying to mathematicians. Axiomatic proof is great for showing things are true in one sense, but it’s not particularly resonant to students. That’s why limits are really foregrounded — because in some abstract sense, all of it is philosophically grounded in limits. But you really don’t have to start with limits and make them the center of everything.

“You don’t have to start with limits and make them the center of everything.”

Ars: I think most people who take calculus find that derivatives are pretty easy — the same process over and over, with a few exceptions that need to be memorized — but integrals are much harder, requiring a kind of intuition, or a bit of guesswork. How do you teach something that is almost an art?

(Orlin) : To me, the dichotomy you’re talking about is one of the most interesting things about calculus: that we have this complete and well-developed theory of derivatives that is quite mechanical. It’s not hard to tell a computer how to take derivatives. With integrals, you can do it in one direction, but trying to do the reverse is very hard. And with derivatives there are maybe eight types of problems that you have to learn, while when it comes to integrals, there are essentially a limitless supply. So how do you teach it? I think it just takes a lot of patient practice.

There’s an event called theMIT Integral Bee, founded by a professor at Harvey Mudd namedAndy Bernoffwhen he was an undergrad at MIT around 1980. Integrals are like English spellings, where they have a hundred different etymologies, and two that sound identical can actually be spelled very differently. Similarly, integrals that look almost identical might have very different solutions, so they have this fun puzzle-y aspect to them.

Bernoff’s observation is that integral skills have gotten a lot worse over the last 30 – 40 years for probably the same reason that our spelling has gotten a lot worse: we don’t need it anymore. Computers are pretty good at fixing it. I think understanding the limitations of our theory of integrals is important, but learning a lot of the integration techniques maybe aren’t worth such a featured spot in our secondary math curriculum.

Ars: Therehave been callsabout revamping high school mathematical curricula to teach statistics, for example, asopposed to algebraand calculus. What are your thoughts on that?

(Orlin) : The questions are fair. And they demand that we reawaken our sense of the beauty and power of calculus. I think the different subjects accomplish different things. The kind of thinking you do in a calculus course is different from the kind of thinking you do in a good statistics course. I definitely don’t like the idea of ​​abolishing calculus. But I taught in the UK for a few years, where there are not separate courses for algebra and geometry and calculus and so on. It’s an integrated math curriculum. Most countries work that way. You could move more of the math education into other subjects that are currently users of mathematics, like physics. In the UK, at least, the university system tends to work this way. If you’re majoring in the sciences, you’ll have math courses taught by the scientists. You won’t go to the math department to take those courses.

There’s something peculiar about the US where we stratify math. We carve out math as a separate discipline, with its own instructional approach. The US system winds up putting calculus on this pedestal as the culmination of secondary math education, when really, yes, it’s a beautiful field of math, but it’s just one important thread in a larger tapestry.

Ars: One last question: how would you make the case for calculus to someone who is skeptical of its relevance, and maybe even a little math-phobic?

(Orlin) : Calculus takes the most vexing and mysterious things imaginable — motion, change, the flow of time — and boils them down to ironclad rules of computation. It takes this world of flux, which is totally ineffable, and reduces it to symbolic procedures and equations you can solve mechanically. That’s a kind of magic that has inspired thinkers for generations. It inspired[Leo] Tolstoy,[Jorge Luis] Borges, andDavid Foster Wallace. It shaped visions of history, ethics, and the powers of the human mind. Calculus is the canonical example of turning the impossible into the routine, and its ideas have nourished not only science, but economics, philosophy, and even literature, too.

That’s the case I wanted to make in this book. Not a defense of calculus as it’s currently taught — heaven knows we could do better — but an exploration of the human side of calculus, what it has meant over the years to everyone from scientists to poets to philosophers to dogs. If calculus is going to remain a fixture of math education — even for those not pursuing STEM careers — then we need to bring out its humanity, to find a version of calculus that speaks to everyone.